

 Share This Page
Share This Page| Home | | Mathematics | |  |  |  Share This Page Share This Page |
A concise summary of trigonometric equations.
Copyright © 2009, Paul Lutus — Message Page
Right Triangle Element Names:
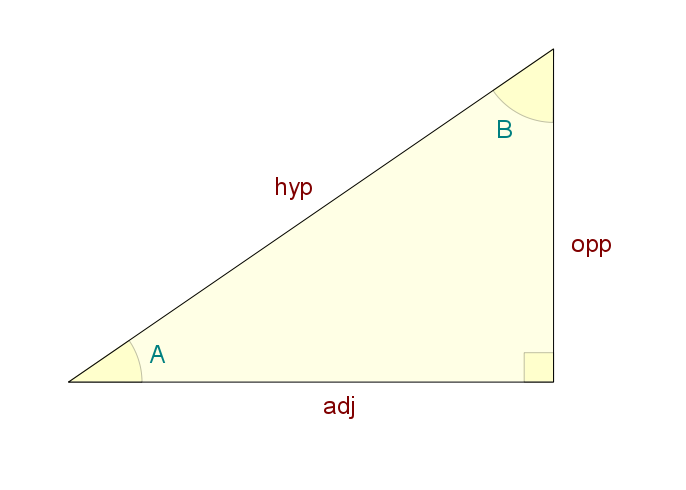
Relationship Matrix (angles in radians):
H |
|
Notes:
$ \displaystyle \frac{\pi}{2} \text{radians} = 90^{\circ}$
$ \displaystyle \sin(A) = \frac{opp}{hyp}$
$ \displaystyle \cos(A) = \frac{adj}{hyp}$
$ \displaystyle \tan(A) = \frac{opp}{adj}$
$ \displaystyle \sin(B) = \frac{adj}{hyp}$
$ \displaystyle \cos(B) = \frac{opp}{hyp}$
$ \displaystyle \tan(B) = \frac{adj}{opp}$
References:
| Home | | Mathematics | |  |  |  Share This Page Share This Page |